
Differential Calculus cuts something into small pieces to find how it changes. Basic calculus explains the two different calculus types called Differential Calculus and Integral Calculus. The word Calculus comes from Latin meaning 'small stone', Because it is like understanding something by looking at small pieces. Some concepts, like continuity, exponents, are the root of advanced calculus. Both concepts have been based on the idea of limits and functions. Symbolic Logic I: The Propositional Calculus. Basic Calculus is the study of the differentiation and integration. Encyclopaedia of Mathematics: Monge-Ampère Equation - Rings and Algebras. Symbolic Logic and Mechanical Theorem Proving.
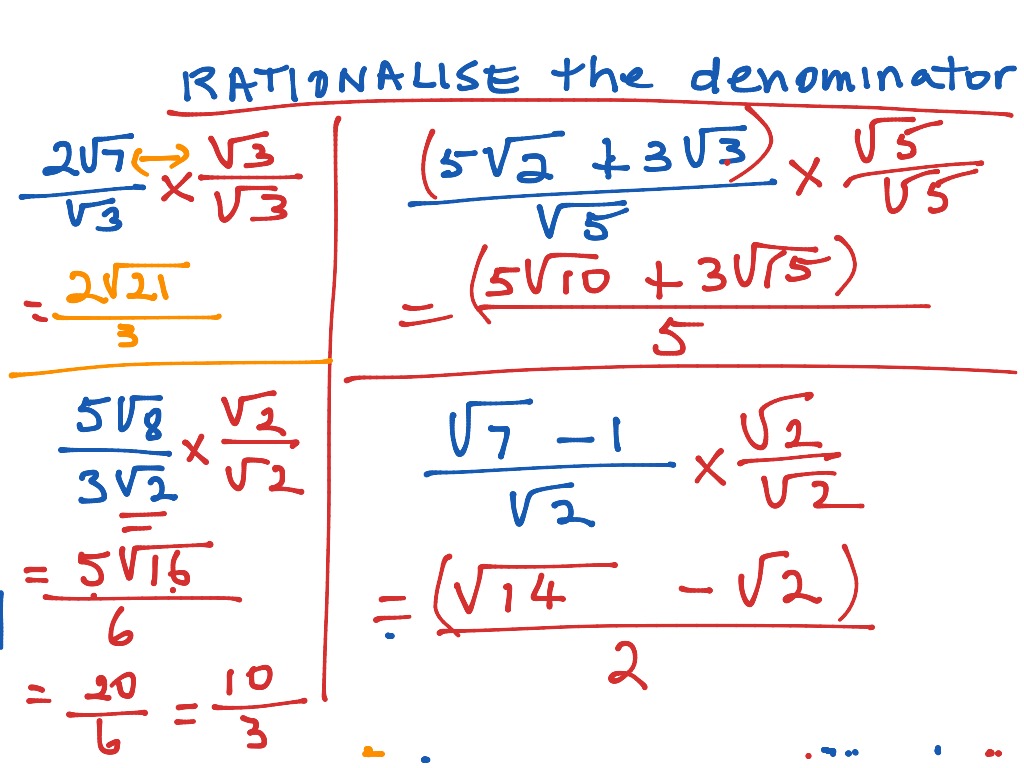
“Dogs” and “Tails” are the terms of the statement, which have the same role in logic as nouns and pronouns in language. f ( x) 5 is a horizontal line with a slope of zero, and thus its derivative is also zero. These include the constant rule, power rule, constant multiple rule, sum rule, and difference rule. Newton’s method is a technique that tries to find a root of an equation. “All dogs have tails” is an example of a predicate-used to describe properties or relationships between individuals or objects. Some differentiation rules are a snap to remember and use. The physical, graphical, algebraic and geometric interpretations of basic ideas of Calculus are explained in such great detail that students can look at Calculus from a refreshing viewpoint and dispel the impression that it is a tough subject. The object of predicate calculus, a generalization of propositional calculus, is to identify individuals, along with their predicates and properties.Īs an example, the following argument cannot be expressed using propositional calculus, but it can be expressed with predicate calculus (ari, n.d.):įrom these sentences, you could conclude that Butch (the individual) has a tail. Predicate Calculus is a more complex version, allowing relations, quantifiers, and functions of any number of variables (Goldmakher, 2020). This calculi forms the basis of the majority of logical-mathematical theories Many complex problems can be reduced to a simple propositional calculus statements, making them easier to solve (Hazelwinkel, 2013). The following are not propositional statements, because they don’t have a clear true/false answer, or have a subjective answer: King Henry VIII had sixteen wives (False).


 0 kommentar(er)
0 kommentar(er)
